Service & Technology
What is microwave heating
It is estimated that the chemical industry accounts for around 40% of the Japanese manufacturing sector’s total energy consumption. Within that industry, particularly large amounts of energy are consumed in operations that involve heating, such as reactions, cracking, distilling, and drying.
In these heating operations, energy is transferred via the transmission of thermal energy, which occurs in three forms: thermal conduction, convection, and radiation. Thermal conduction is a phenomenon whereby heat is transmitted inside a solid or stationary fluid, from the hot side to the cold side. One example of this from the chemical industry is when a heat transfer medium such as steam passes through a heat exchanger or jacketed reactor: the heat is transferred to the vessel itself and its temperature rises. Convection refers to heat being transferred by the movement of fluids. Examples include solid bodies being heated by blowing hot air onto them, or boilers where heat is transferred by the actual substance being heated receiving thermal energy from a heat transfer surface and moving.
Radiation occurs when thermal energy is transmitted through space by infrared rays (which, like microwaves, is a type of electromagnetic waves). An example of this is a naphtha cracking furnace for ethylene production, which makes use of radiant heat from the flames when gas is combusted.
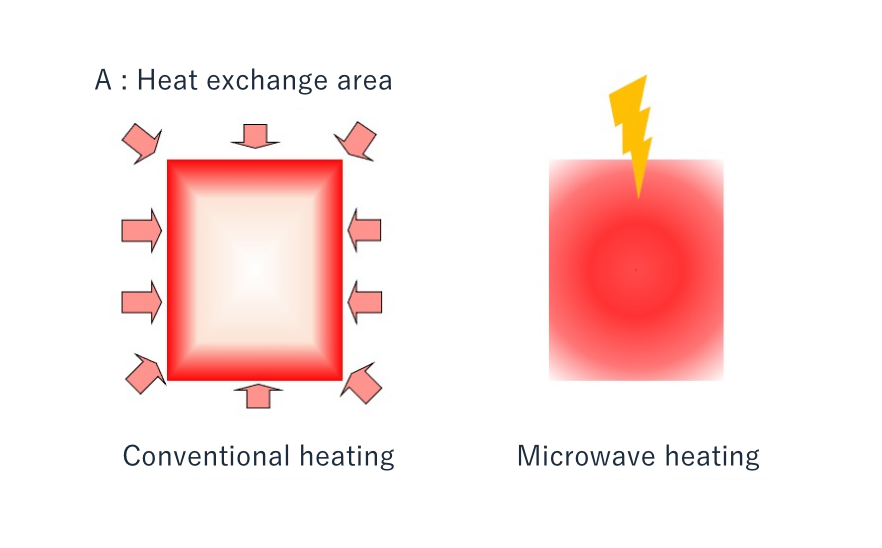
All the above methods share the feature that the transfer of thermal energy requires a heat source hotter than the object to be heated. And this must be done either via a vessel holding the material to be heated (examples include a liquid passing through a heat exchanger, a liquid in a reactor, or a fluid passing through the tube of a cracking furnace), or via direct contact with a fluid heat source. We refer to these methods as ‘conventional’ heating.
Microwave heating, in contrast, is a method that uses a completely different means of energy transfer. Microwaves are electromagnetic waves, propagating through changes in electric / magnetic fields. Electromagnetic waves are classified according to their frequency (wavelength) into x-rays, ultraviolet rays, visible light, infrared rays, and radio waves. Radio waves which have frequencies between 300 MHz and 300 GHz (or wavelengths of between 1 m and 1 mm) are known as microwaves.
Microwaves can transfer energy directly to dielectrics*, so the actual object being heated behaves like the heat sources in conventional heating. This means that unlike conventional heating, there is no need for a heat source hotter than the object to be heated, which in turn means that the processes and equipment can be expected to be more effective than those of the past.
* The IEC defines microwave heating as “to heat dielectric materials by generating heat through mainly their molecular motion and their ionic conduction”. In reality, dielectrics are not the only things that can be heated by microwave, but here we are considering microwave heating in its narrow sense.

Comparison with conventional heating
Heat transfer volume for conventional heating
As an example, let’s consider the jacketed reactors commonly used in chemical plants. With conventional methods, thermal energy from a heat medium (e.g., steam or hot oil) passing through the jacket is transferred to the walls of the reaction vessel by convection. This heat is then transferred to the interior vessel wall by conduction, and finally transferred to the liquid inside by means of convection again. The heat transfer per unit time under these conditions, Q[W], can be expressed by the following equation (1):
Q = U・A・Δt …(1)
Here, U is the overall heat transfer coefficient (W/m2・K), a measure of the ease of heat transfer. Its magnitude depends on the reactor material and movement of the fluids. A is the heat transfer area (m2). In this case, it refers to the surface area of the parts covered by the jacket, where heat transfer can take place. ΔT is the temperature difference between the heat source and the object to be heated (K). This generally varies depending on the position of the container, so a logarithmic mean is often used as an approximation.
As can be seen from equation (1), supplying a great amount of thermal energy to the reactor necessitates increasing U, A or ΔT (or indeed all of them). Examining U first, it is a coefficient that depends on the material and the movement of the fluids and changing it significantly would lead to changes in the process itself, so is generally not a simple task. So, all one needs to do is increase A? It’s not that easy. That’s because the capacity of the reactor is generally determined by the production volume, and consequently the heat transfer area A is also fixed within a certain geometric range. It is simply not possible to increase the heat transfer area A without limit, in isolation. This is particularly true as the process scales up, since the ratio of surface area to volume decreases proportionately. So, we are left with ΔT. But to increase this, the temperature of the heat medium must be increased, and higher temperature heat sources are usually more costly, so it is not always easy to obtain the required temperature. Moreover, raising the temperature too high can also lead to other problems such as thermal degradation and other side effects.
Heat transfer volume of microwave heating
Let us now compare that with microwave heating. The energy supplied by microwave dielectric heating is expressed by the following equation (2).
P = α・ε”・E2 …(2)
Here, P is the calorific value per unit volume (W/m2). a is a constant, and ε″ is a material-specific value called the dielectric loss factor. E is the strength of the electric field (V/m) produced by the microwave, that acts on the material.
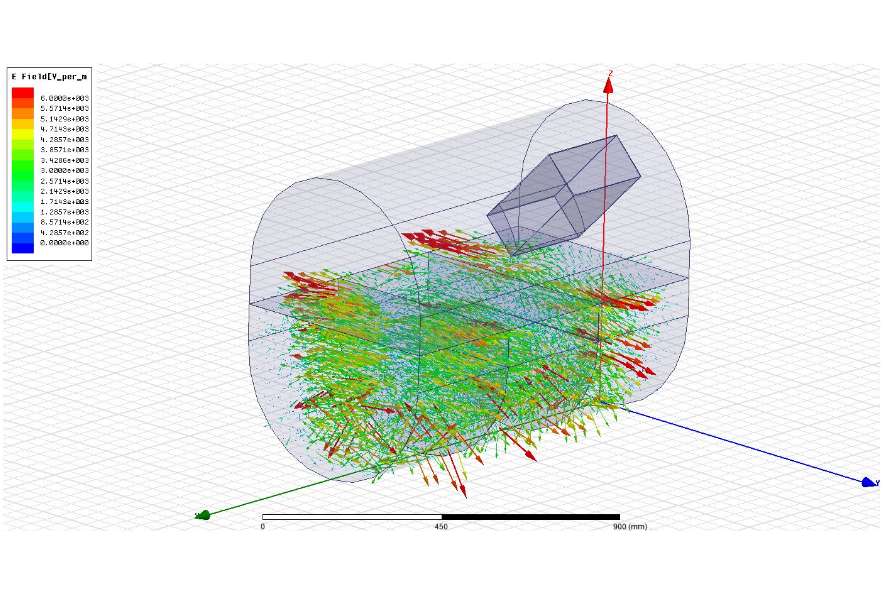
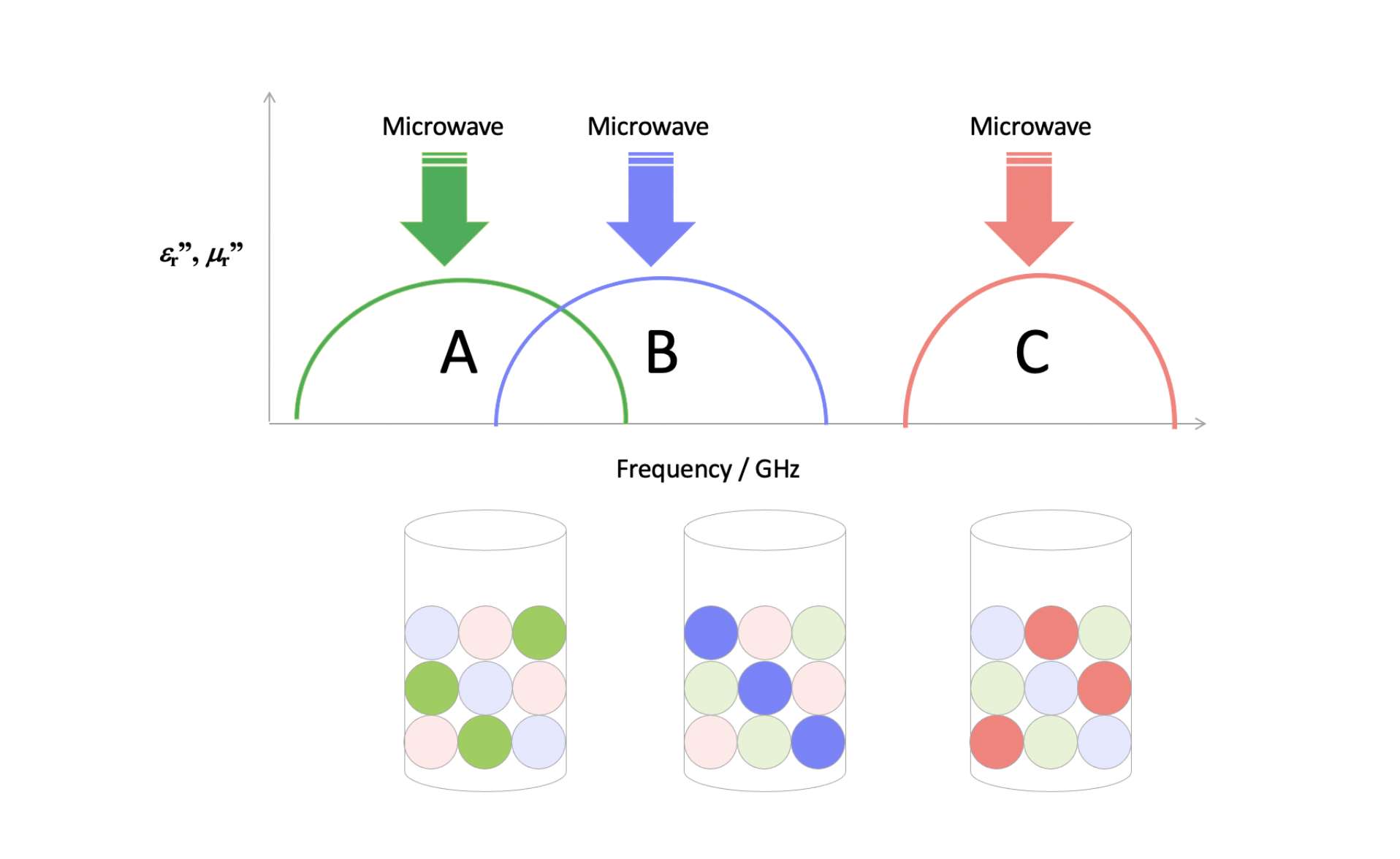
Equation (2) shows that the amount of energy that can be transferred is related to the intrinsic microwave absorption capacity ε″ of the material being heated, and the strength of the electric field strength acting on it (E). There is no direct relation to A or ΔT, which are so important in conventional heating, and this can therefore provide an advantage with respect to scaling up or securing high-temperature heat sources, which are challenges for conventional heating methods. The ε″ of the material to be heated is substance-specific, but does vary with temperature and frequency, so these effects also need to be taken into account when gathering data. Electromagnetic field analysis based on suitable data (to calculate the field strength distribution) makes it possible to estimate P correctly in the above equation. One of MWCC’s strengths is that we have measured and accumulated data on a wide range of substances at various temperatures and frequencies.
Electromagnetic field analysis
Having described the features of conventional heating and microwave heating, let us now compare the two.
If we divide Q in equation (1) by the volume V of the device parts which contribute to heating, the units become W/m3. This is the same unit as for P in equation (2), which allows for a direct comparison. In conventional heating, substances other than the target material have to be heated at the same time, so V ends up larger than the volume of the target material, and in most cases Q/V<P. This means that microwave heating has a higher calorific value per unit volume (W/m3) and can be a more “efficient” heating method. More “efficient” means smaller equipment, shorter processing times and less heat loss (lower energy consumption).
Benefits of microwave heating
One of the potential advantages of microwave heating is that it is more efficient than conventional heating, as explained above, but there are many other possible benefits. For example, no temperature difference is required for this form of heating, thus eliminating the need for extensive equipment to create a high-temperature heat source. A further potential benefit is the option of selective heating. By selecting the right frequency, microwave heating can in heterogeneous systems transfer energy directly to the target reaction material, rather than the solvent; or in cases where a multilayer film contains a layer to be heated, it can heat only that target layer. If, as a consequence, one can anticipate lower energy consumption or higher quality, then microwave heating is worth considering as an alternative to conventional methods.
As noted at the outset, the chemical industry makes use of a range of heating operations, and MWCC has a proven track record of applying microwaves to these, to achieve efficient energy supply processes.
We believe that energy consumption in the chemical industry can be significantly reduced by promoting this technology.
Here is a list of benefits we have identified in our work to date:
- Shorter reaction and processing times
- Lower energy consumption (and CO2 emissions)
- Smaller equipment (saving space)
- Higher yields (fewer raw materials)
- Greater quality (purity, color, etc.)
- Reduced catalyst quantities
- Production of new substances that cannot be made with conventional heating method
If you are now wondering whether microwaves could be applied to your own processes and lead to innovative results, please do not hesitate to get in touch!